Minimizing Cost Shipping Strategy

ARE YOU LOOKING FOR RELIABLE MINIMIZING COST SHIPPING STRATEGY ASSIGNMENT HELP SERVICES? EXPERTSMINDS.COM IS RIGHT CHOICE AS YOUR STUDY PARTNER!

Question: Develop your capability in applying your skills and knowledge in a hypothetical situation and to build your problem solving and report writing skills.

Answer: Problem Formulation: The main objective of this study is to determine an optimal solution to minimize the shipping cost. In this study, the company taken into consideration produces mandarins at three plants (named as Plant 1, Plant 2 and Plant 3) which will be either shipped directly to customers (two customers are listed, namely, Customer 1 and Customer 2) or to warehouses (two warehouses, namely, Warehouse 1 and Warehouse 2) from where it was shipped to customers (two customers are listed, namely, Customer 1 and Customer 2). Thus, the main objective of this study is to minimize the shipping cost

Let P₁, P₂ and P₃ represents the three plants from which mandarins are produced and W1 and W2 represents the two warehouses and C₁ and C₂ represents two customers respectively.

P_ij represents mandarins shipped from Plant i to Plant j

Therefore, the objective function is

Minimize Z = 3*P₁₂ + 3*P₁₃ + 6*P₁W₁ + 7*P₁W₂ + 15 * P₁C₁ + 15 * P₁C₂ + 2 *P₂₃ + 3*P₂₁ + 5*P₂W₁ + 6*P₂W₂ + 16 * P₂C₁ + 16 * P₂C₂ + 3*P₃₁ + 2*P₃₂ + 7*P₃W₁ + 6*P₃W₂ + 14 * P₃C₁ + 15 * P₃C₂ + 3*W₁₂ + 6*W₁C₁ + 8 * W₁C₂ + 3*W₂₁ + 7*W₂C₁ + 7 * W₂C₂ + 4 * C₁₂ + 4 * C₂₁

Subject to the constraints

ΣP_1j + ΣP₁W_k + ΣP₁C_k < = 350

ΣP_2j + ΣP₂W_k + ΣP₂C_k < = 400

ΣP_3j + ΣP₃W_k + ΣP₃C_k < = 450

ΣC₂W_k + ΣP_iC₂< = 480

ΣC₂W_k + ΣP_iC₂< = 460

P₁, P₂, P₃, C₁, C₂, W₁ and W₂ > = 0

EXPERTSMINDS.COM GIVES ACCOUNTABILITY OF YOUR TIME AND MONEY – AVAIL TOP RESULTS ORIGINATED MINIMIZING COST SHIPPING STRATEGY ASSIGNMENT HELP SERVICES AT BEST RATES!

Problem Solving: The problem is solved using excel solver and the output screenshot of the workings is given below

Select the cell that contains the objective function and place it in the "Set Objective" box

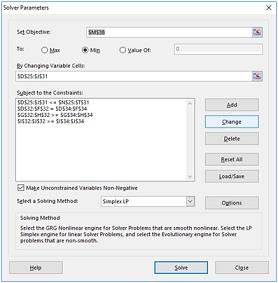
Add the constraints by clicking Add Icon

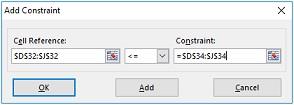
Add the constraints one by one by clicking Add Icon and after adding the last constraints click Ok icon to return back to main menu
In "Select a Solving Method", select the option "Simplex LP" and click Solve

The Solver output is given below

24/7 AVAILABILITY OF TRUSTED MINIMIZING COST SHIPPING STRATEGY ASSIGNMENT WRITERS! ORDER ASSIGNMENTS FOR BETTER RESULTS!

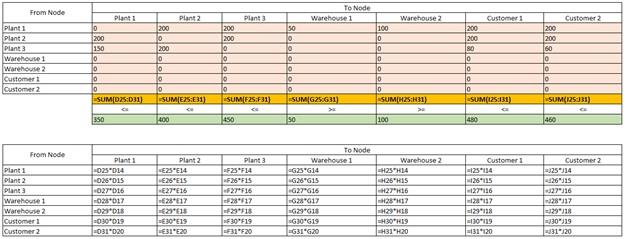
The formula used to frame the linear programing problem is given below

Discussion: This study mainly focus on minimizing the shipping cost to the company that produces mandarins in their three plants.
The simplex linear programming problem method was used to solve this problem and the minimum shipping cost was found to be 18470 (in thousands of dollars)

This minimum shipping cost is obtained by shipping mandarins from

Plant 1 to Plant 2 → 200 mandarins

Plant 1 to Plant 3 → 200 mandarins

Plant 1 to Warehouse 1 → 50 mandarins

Plant 1 to Warehouse 2 → 100 mandarins

Plant 1 to Customer 1 → 200 mandarins

Plant 1 to Customer 2 → 200 mandarins

Plant 2 to Plant 1 → 200 mandarins

Plant 2 to Plant 3 → 200 mandarins

Plant 2 to Customer 1 → 80 mandarins

Plant 2 to Customer 2 → 60 mandarins

Plant 3 to Plant 1 → 150 mandarins

Plant 3 to Plant 2 → 200 mandarins

Thus, the minimum shipping cost is 18470 (in thousands of dollars)

Recommendation: Further the study can be improved by increasing the demand and capacity of the Mandarins which will certainly help the management to use the simulation techniques from which they can have a better idea of their further demands for mandarins.

SAVE DISTINCTION MARKS IN EACH MINIMIZING COST SHIPPING STRATEGY ASSIGNMENT WHICH IS WRITTEN BY OUR PROFESSIONAL WRITER!